Resistance Depends on Area of Cross Section
Resistance Depends on Area of Cross Section
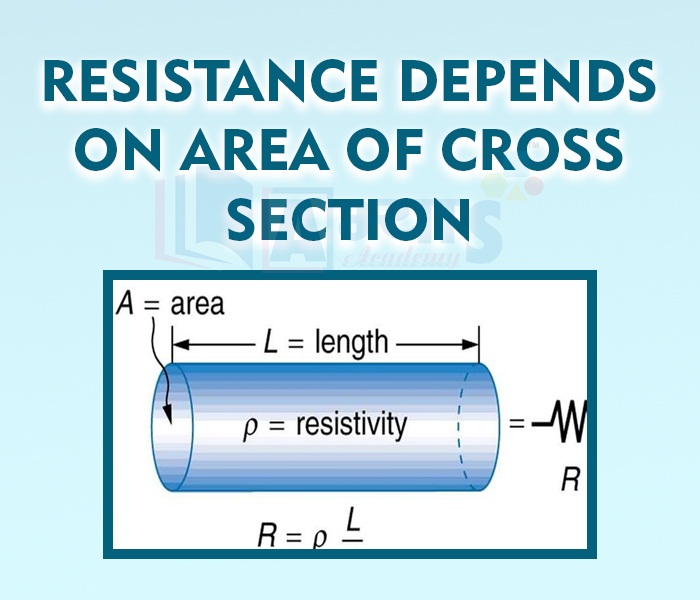
Resistance Depends on the Area of Cross Section of the wire:
The resistance of ohmic circuit elements such as metal wires or carbon resistor depends on the area of cross section of the wire. If we fix the length of the copper wire and vary the thickness or cross-sectional area A then we find the resistance of the copper decreases as the cross-sectional area A of the wire increases. To find dependence,we will follow the following steps
1 Keep the length of the wire as fixed and just increase or decrease the area of cross section of the wire.
2. Apply a potential difference across the ends of the wire and calculate the value of R from the values of current and potential difference
3. Calculate the value of R for different values of the area of cross section of the wire.
4. Plot a graph as shown below by taking the reciprocal of area of cross section along x axis and resistance along y axis
From the first graph we observe that as the reciprocal of area of cross section increases the resistance also increases. So we find that there is a proportionality between the reciprocal of area of cross section A of the wire and the resistance of the wire R. The resistance of a conductor R is directly proportional to the reciprocal of area of cross section
5. We can also plot a graph as shown below by taking the area of cross section along x axis and resistance along y axis
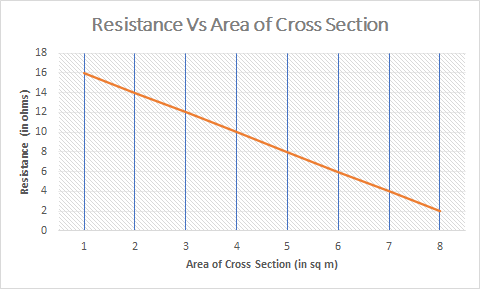
From this graph we observe that as the area of cross section increases the resistance decreases. So we find that there is a proportionality between the area of cross section A of the wire and the resistance of the wire R. The resistance of a conductor R is inversly proportional to the area of cross section
In fact we get an inverse relationship between area of cross section and resistance and it can be stated as
We know that Resistance is directly proportional to the length of the wire. It can be stated that
Combining the expressions of relation of resistance with length and area of cross section of a wire we get
:
To simplify this expression, we can replace the product of the two constants with a single new constant and write
This constant is known as as Resistivity and it depends on the material of the conductor.
Illustration: In a circuit when a current of 2 A flows through a certain wire having resistance R a potential difference of 8 V is generated across the wire. What will be the new potential difference generated across the wire if the area of cross section of the wire is doubled.
Solution: It is given that
V= 8 V
I = 2 A
R= ?
V= IR
As the area of cross section of the wire is doubled so the resistance will be halved because resistance is inversely proportional to area of cross section..
So new potential difference of 4V is generated across the wire if the area of cross section of the wire is doubled.
Illustration: Calculate the resistance of a conductor when its length is doubled
Solution: We are given that the length is doubled so
Graph as showing reciprocal of area of cross section along x axis and resistance along y axis will be a ______________________ .
| |||
| Right Option : A | |||
| View Explanation | |||
If the length of a wire is 3 km and its diameter is 2 cm, then calculate its resistance.
| |||
| Right Option : B | |||
| View Explanation | |||
The resistance of a thin wire in comparison of thick wire of the same meterial - | |||
| Right Option : D | |||
| View Explanation | |||
Students / Parents Reviews [20]
One of the best institutes to develope a child interest in studies.Provides SST and English knowledge also unlike other institutes. Teachers are co operative and friendly online tests andPPT develope practical knowledge also.
Aman Kumar Shrivastava
10thThe experience was nice. I studied here for three years and saw a tremendous change in myself. I started liking subjects like English and SST which earlier I ran from. Extra knowledge gave me confidence to overcome competitive exams. One of the best institutes for secondary education.
Aman Kumar Shrivastava
10thMy experience was very good with Abhyas academy. I am studying here from 6th class and I am satisfied by its results in my life. I improved a lot here ahead of school syllabus.
Ayan Ghosh
8thAbhyas institute is one of the best coaching institute in the vicinity of Ambala cantt.The institute provides good and quality education to the students.The teachers are well experienced and are very helpful in solving the problems. The major advantages of the institute is extra classes for weak...
Shreya Shrivastava
8thWhen I have not joined Abhyas Academy, my skills of solving maths problems were not clear. But, after joining it, my skills have been developed and my concepts of science and SST are very well. I also came to know about other subjects such as vedic maths and reasoning.
Sharandeep Singh
7thThird consective year,my ward is in Abhyas with nice experience of admin and transport support.Educational standard of the institute recumbent at satisfactory level. One thing would live to bring in notice that last year study books was distributed after half of the session was over,though study ...
Ayan Ghosh
8thWe started with lot of hope that Abhyas will help in better understnding of complex topics of highers classes. we are not disappointed with the progress our child has made after attending Abhyas. Though need to mention that we expected a lot more. On a scale of 1-10, we would give may be 7.
Manya
8thIt was a good experience with Abhyas Academy. I even faced problems in starting but slowly and steadily overcomed. Especially reasoning classes helped me a lot.
Cheshta
10thMy experience with Abhyas Academy has been very good. When I was not in Abhyas whenever teacher ask questions I could not speak it confidently but when I came in Abhyas, my speaking skills developed and now I am the first one to give the answer of teachers question.
Upmanyu Sharma
7thAbhyas is a complete education Institute. Here extreme care is taken by teacher with the help of regular exam. Extra classes also conducted by the institute, if the student is weak.
Om Umang
10thAbhyas is good institution and a innovative institute also. It is a good platform of beginners.Due to Abhyas,he has got knoweledge about reasoning and confidence.My son has improved his vocabulary because of Abhyas.Teacher have very friendly atmosphere also.
Manish Kumar
10thAbhyas is an institute of high repute. Yogansh has taken admission last year. It creates abilities in child to prepare for competitive exams. Students are motivated by living prizes on basis of performance in Abhyas exams. He is satisfied with institute.
Yogansh Nyasi
7thBeing a parent, I saw my daughter improvement in her studies by seeing a good result in all day to day compititive exam TMO, NSO, IEO etc and as well as studies. I have got a fruitful result from my daughter.
Prisha Gupta
8thAbout Abhyas metholodology the teachers are very nice and hardworking toward students.The Centre Head Mrs Anu Sethi is also a brilliant teacher.Abhyas has taught me how to overcome problems and has always taken my doubts and suppoeted me.
Shreya Shrivastava
8thAbhyas academy is great place to learn. I have learnt a lot here they have finished my fear of not answering.It has created a habit of self studying in me.The teachers here are very supportive and helpful. Earlier my maths and science was good but now it has been much better than before.
Barkha Arora
10thUsually we see institutes offering objective based learning which usually causes a lag behind in subjective examinations which is the pattern followed by schools. I think it is really a work of planning to make us students grab the advantages of modes of examination, Objective Subjective and Onli...
Anika Saxena
8thAbhyas Methodology is very good. It is based on according to student and each child manages accordingly to its properly. Methodology has improved the abilities of students to shine them in future.
Manish Kumar
10thIn terms of methodology I want to say that institute provides expert guidence and results oriented monitering supplements by requsite study material along with regular tests which help the students to improve their education skills.The techniques of providing education helps the students to asses...
Aman Kumar Shrivastava
10thMy experience with Abhyas academy is very nice or it can be said wonderful. I have been studying here from seven class. I have been completing my journey of three years. I am tinking that I should join Abhyas Academy in tenth class as I am seeing much improvement in Maths and English
Hridey Preet
9thMy experience with Abhyas academy is very good. I did not think that my every subject coming here will be so strong. The main thing is that the online tests had made me learn here more things.
